Equilateral triangles, those perfectly symmetrical wonders, have tickled mathematicians and artists alike for centuries. From ancient Egyptian pyramids to modern-day art installations, these triangles have been omnipresent.
Did you know that their internal angles always sum up to a precise 180 degrees? Or that their appearance in color theory has influenced countless artists? Get ready to uncover fun facts about equilateral triangles that’ll reshape the way you see this three-sided marvel.
1. Ancient Obsession: Triangles in Pyramids
The ancient Egyptians were geometry aficionados! The majestic pyramids of Giza, built around 2580-2560 BCE, are a testament to their obsession with the equilateral triangle. The sides of these pyramids are vast equilateral triangles, serving both an aesthetic and structural purpose. Historians believe that the equilateral triangle’s equal sides and angles symbolized harmony and the cycle of life for the Egyptians.
This isn’t just a coincidence; it’s a conscious design backed by a deep understanding of geometry. These structures have withstood the test of time, and the equilateral triangle’s properties play a significant role in their resilience.
2. The Equiangular Enigma: Three Angles, Three Sixties
Beyond its equal sides, the equilateral triangle is equally fascinating when you delve into its angles. Every angle in an equilateral triangle measures exactly 60 degrees. This makes it not only equilateral but also equiangular. Mathematicians have been intrigued by this perfect trifecta of angles for centuries.
Historically, this angle fact was used in various calculations, especially in navigation. Mariners often employed tools like the astrolabe, which incorporated the principles of equilateral triangles, to help navigate and measure the altitude of stars.
3. Congruent Comrades: All Sides Have Equal Length
Congruency is the beating heart of the equilateral triangle. Every side, without fail, matches its counterpart in length, providing this geometric wonder with its iconic look. Did you know that the word ‘equilateral’ is derived from Latin? “Equi” means ‘equal’ and “latus” stands for ‘side’. It’s no wonder this triangle is known for its equal length sides!
In the 5th century BC, the Greek mathematician Hippasus showed that equilateral triangles are the only triangles where the sides are congruent. Thanks to his discovery, it became a foundation of Euclidean geometry studies in academia.
4. Symmetry Supreme: The Equilateral’s Three Axes
One of the mesmerizing properties of the equilateral triangle is its sublime symmetry. Not one, not two, but three lines of symmetry can be drawn through this triangle, each passing through a vertex and bisecting the opposite side. This inherent symmetry has made the equilateral triangle a go-to choice for artists and architects throughout history.
In the Renaissance period, architects praised the equilateral triangle for its balance and used it frequently in cathedrals and frescoes. Its threefold symmetry represented the Holy Trinity, adding a layer of spiritual significance to its geometric charm.

Image: Wikimedia Commons
5. Definition Distinction: Equilateral vs. Isosceles Triangle
Ah, the age-old debate! While both these triangles boast of equal sides, the equilateral triangle takes it up a notch with all three sides being identical. An isosceles triangle, on the other hand, settles with just two sides of equal length. The ancient Greeks were among the first to rigorously define and distinguish between these two.
Aristotle, in his work, referenced the distinctions between these triangles, drawing philosophical analogies. It’s not just about lines and angles; it’s about understanding the essence of equality and how it varies in different contexts.
6. Geometry Gold: Area and Perimeter Formula
When it comes to formulas, the equilateral triangle doesn’t shy away! With side length ‘a’, the area can be calculated using the formula: (sqrt(3)/4) × a^2. As for the perimeter? Simply 3a. These formulas have been fundamental in geometry since the days of ancient scholars.
The renowned mathematician Euclid, in his seminal work “Elements”, laid down the principles and formulas concerning equilateral triangles, which have since been the cornerstone of geometry. Through centuries, these formulas have been employed in various fields, from construction to computer graphics.
7. In Real Life: Equilateral Triangles in Nature
Nature is quite the mathematician, and the equilateral triangle is one of her favorite shapes. If you’ve ever stared in wonder at the honeycomb in a beehive, you’ve witnessed equilateral triangles in all their natural glory. Bees, being the efficient builders they are, use this shape because it allows them to use the least amount of wax while storing the maximum amount of honey.
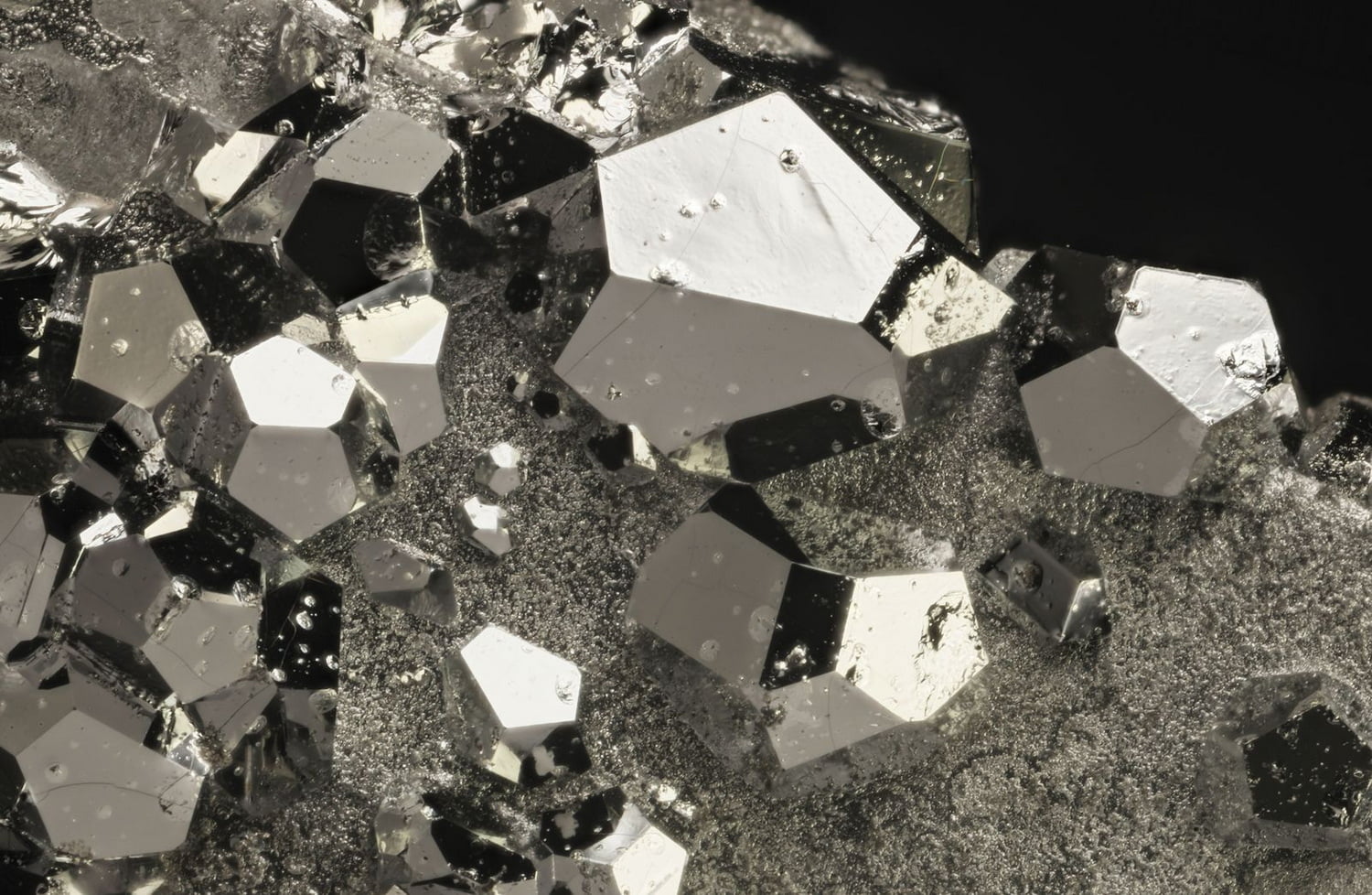
The crystalline structure of certain minerals, like those in quartz, often showcases an intricate arrangement of equilateral triangles. It’s truly a testament to how this geometric shape is a fundamental part of the universe’s real life design.

David Hablützel / Pexels
8. Right Triangle’s Sibling: Not Always a Right Angle!
Equilateral triangles seem to enjoy keeping us on our toes! Contrary to the right triangle, equilaterals don’t have a 90-degree angle. Instead, they boast three identical angles of 60 degrees each. It’s a common misconception, especially among geometry newcomers, to mix up the two.
While right triangles and their famous Pythagorean theorem have their unique charm, equilateral triangles come with their set of quirks, ensuring their place in the hall of fame of the triangle family.
9. Properties Power: The Inradius and Circumradius Connection
Equilateral triangles pack a punch with their fascinating properties. Dive deep, and you’ll discover the connection between their inradius (radius of the inscribed circle) and circumradius (radius of the circumscribed circle). For a triangle with side length ‘a’, the inradius is a/(2√3) and the circumradius is a/√3. The beauty lies in the relationship: the circumradius is exactly twice the inradius!
Historical records indicate that ancient Indian mathematicians were well aware of this relationship. Their works on circle-triangle interrelationships set the stage for many geometric explorations in later centuries.
10. Tessellation Temptation: A Perfect Fit
Tessellation, the art of covering a surface with a pattern of flat shapes so that there are no overlaps or gaps, finds a loyal friend in the equilateral triangle. These triangles can fit together seamlessly to cover a plane without any spaces in between. It’s no surprise that they are a favorite choice for many tile designs and artworks.
M.C. Escher, the famous graphic artist, used equilateral triangle tessellations in some of his iconic works. It’s amazing how a simple geometric shape can transform into a canvas of infinite possibilities in the hands of a creative genius.
11. Degrees of Delight: Summing Up to 180
Triangles have this cool party trick: no matter their shape or size, the sum of their internal angles always add up to a neat 180 degrees. Equilateral triangles split this sum equally among their three angles, giving each angle a value of 60 degrees. This degrees of consistency is quite remarkable!
Historians believe that by 1800 BC, ancient Egyptians had a decent understanding of this triangle angle sum concept. They applied this knowledge in various construction projects, including their iconic pyramids.

Image by wirestock / Freepik
12. The Altitude Adventure: It’s a Median Too!
The equilateral triangle never ceases to surprise. Draw an altitude (a line segment from a vertex and perpendicular to the opposite side) in one, and bam! You’ve also drawn a median (a line segment from a vertex to the midpoint of the opposite side). In equilateral triangles, the altitude and median are the same line!
This geometric revelation dates back to the Greeks. They revered geometry, and discoveries like this added to the mystique of equilateral triangles in their scholarly pursuits.
13. Trivia Time: World Records and Equilateral Triangles
Ever heard of the world record for the largest human-formed equilateral triangle? In 2010, over 6,000 students in India formed an equilateral triangle, highlighting the fun fact that geometry can be both educational and entertaining!
Equilateral triangles have also found their place in Guinness World Records for various feats, including largest structures and designs based on this shape. They’re not just classroom wonders but also record-breaking stars!
14. The Equilateral in Pop Culture: Art and Design Marvel
Equilateral triangles aren’t just for mathematicians; they’ve been muses for artists and designers too. Their symmetrical beauty has graced album covers, posters, and even film sequences. In the 1960s and 70s, equilateral triangles became symbols of futuristic design, prominently featured in sci-fi art and literature.
Famous artists like Andy Warhol have incorporated equilateral triangles in their pieces. They embody a blend of simplicity and complexity that resonates with both the artist and the observer.

Image: asrarchowdhury.wordpress.com
15. Chromatic Charm: Equilateral Triangle in Color Theory
When artists and designers dive into color theory, the equilateral triangle has a pivotal role to play. Ever glanced at a color wheel? You might’ve noticed that the primary colors (red, blue, yellow) or the secondary colors (green, orange, purple) are often positioned at the vertices of an equilateral triangle. This equilateral triangle fact demonstrates the equal distance between these colors in terms of color harmony.
Historically, Sir Isaac Newton, when he was conceptualizing the color wheel in the 17th century, played with the positioning of colors in triangular formats. It’s a testament to the equilateral triangle’s enduring influence, even in the world of color!
FAQ
Is an equilateral triangle unique?
Absolutely! An equilateral triangle is unique because it’s the only type of triangle where all three sides and all three angles are congruent. While other triangles might have one or two sides of equal length, only in an equilateral triangle are all three sides and angles identical. This uniformity gives it many of its distinctive properties, setting it apart from other triangles.
What is an equilateral triangle also known as?
An equilateral triangle is also commonly known as an “equiangular triangle.” This name stems from its nature of having all three internal angles equal, each measuring 60 degrees. So, while “equilateral” emphasizes its equal sides, “equiangular” highlights its equal angles. Both terms are correct and aptly describe the triangle’s attributes.
Are equilateral triangles the strongest?
In engineering and architecture, triangles are considered the strongest shape due to their inherent stability. Among triangles, the equilateral triangle offers a particular advantage because of its uniformity. When forces are applied to its vertices or sides, the stresses are distributed evenly across its structure. However, the term “strongest” can be subjective and might depend on the specific context or application.
Are all equilateral triangles always similar?
Yes! All equilateral triangles are always similar. Similarity in geometry refers to the condition where two shapes have the same shape but not necessarily the same size. Since equilateral triangles always have angles of 60 degrees at each vertex and three congruent sides, any two equilateral triangles, regardless of their size, will be similar to each other.
Is an equilateral triangle always equilateral?
Yes, by definition, an equilateral triangle is always equilateral. This means that all its sides are of equal length. If a triangle has one or two sides of different lengths, it cannot be termed “equilateral.” The term “equilateral” is unwavering in its definition, denoting equal length in all sides of the triangle.
How many properties does an equilateral triangle have?
Equilateral triangles have a myriad of properties, thanks to their symmetrical nature. Some of the main properties include:
- All three sides are of equal length.
- All internal angles measure 60 degrees.
- The altitude and median drawn from a vertex coincide.
- The inradius and circumradius have a fixed relationship based on the triangle’s side length.
- They can tessellate a plane without gaps.
And the list goes on! Depending on how in-depth you delve into geometry, you can discover numerous properties and characteristics unique to equilateral triangles.